IASbaba’s Daily CSAT Practice Test
ARCHIVES
Daily CSAT Practice Test
Everyday 5 Questions from Aptitude, Logical Reasoning, and Reading Comprehension will be covered from Monday to Saturday.
Make the best use of the initiative. All the best!
To Know More about Ace the Prelims (ATP) 2021 – CLICK HERE
Important Note:
- Don’t forget to post your marks in the comment section. Also, let us know if you enjoyed today’s test 🙂
- After completing the 5 questions, click on ‘View Questions’ to check your score, time taken and solutions.
Test-summary
0 of 5 questions completed
Questions:
- 1
- 2
- 3
- 4
- 5
Information
To view Solutions, follow these instructions:
- Click on – ‘Start Test’ button
- Solve Questions
- Click on ‘Test Summary’ button
- Click on ‘Finish Test’ button
- Now click on ‘View Questions’ button – here you will see solutions and links.
You have already completed the test before. Hence you can not start it again.
Test is loading...
You must sign in or sign up to start the test.
You have to finish following test, to start this test:
Results
0 of 5 questions answered correctly
Your time:
Time has elapsed
You have scored 0 points out of 0 points, (0)
| Average score |
|
| Your score |
|
Categories
- Not categorized 0%
| Pos. | Name | Entered on | Points | Result |
|---|---|---|---|---|
| Table is loading | ||||
| No data available | ||||
- 1
- 2
- 3
- 4
- 5
- Answered
- Review
-
Question 1 of 5
1. Question
A bag contains 6 red and 4 blue balls. 2 balls are drawn one by one without replacement. What is the probability that the balls are alternately of different colors?
Correct
Solution (a)
There are two alternatives that will satisfy the given condition.
Either it can red ball followed by blue balls or it could be blue balls followed by red balls.
So, the required probability will be the sum of two mutually exclusive events.
= p(red ball followed by blue balls) + p(blue balls followed by red balls)
= (6/10 * 4/9) + (4/10 * 6/9) = 8/15
Incorrect
Solution (a)
There are two alternatives that will satisfy the given condition.
Either it can red ball followed by blue balls or it could be blue balls followed by red balls.
So, the required probability will be the sum of two mutually exclusive events.
= p(red ball followed by blue balls) + p(blue balls followed by red balls)
= (6/10 * 4/9) + (4/10 * 6/9) = 8/15
-
Question 2 of 5
2. Question
A is the mother of B. B is the sister of C. D is the son of C. E is the brother of D. F is the mother of E. G is the granddaughter of A. H has only two children B and C.
How F related to H?
Correct
Solution (b)
From the above data it is clear that F is the daughter-in-law of H.
Incorrect
Solution (b)
From the above data it is clear that F is the daughter-in-law of H.
-
Question 3 of 5
3. Question
An office consists of 520 employees working in different departments HR, IT, Production and Marketing. The ratio of men to women in the organization is 5:3. 20 per cent of the men work in the IT department. 40 per cent of the women work in the HR department. The total number of employees in the Production department is 135. 40 percent of the men work in the Production department. Two-fifths of the women work in the IT department and the remaining work in the Marketing department. Four percent of the men work in the HR department and the remaining work in the Marketing department.
The number of men working in the Marketing department forms what percent of the total number of employees in the organization?
Correct
Solution (a)
Ratio of men to women in the organization is 5: 3
Total number of Employee in the organization = 520
Number of Men in the organization = 5/8*520 = 325
Number of women in the organization = 3/8*520 = 195
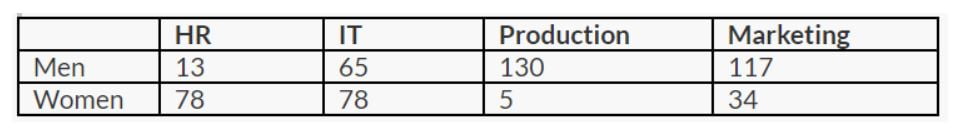
The number of men working in the Marketing department = 117
The total number of employees in the organization = 520
Required percentage = 117/520*100 =22.5%
Incorrect
Solution (a)
Ratio of men to women in the organization is 5: 3
Total number of Employee in the organization = 520
Number of Men in the organization = 5/8*520 = 325
Number of women in the organization = 3/8*520 = 195

The number of men working in the Marketing department = 117
The total number of employees in the organization = 520
Required percentage = 117/520*100 =22.5%
-
Question 4 of 5
4. Question
An office consists of 520 employees working in different departments HR, IT, Production and Marketing. The ratio of men to women in the organization is 5:3. 20 per cent of the men work in the IT department. 40 per cent of the women work in the HR department. The total number of employees in the Production department is 135. 40 percent of the men work in the Production department. Two-fifths of the women work in the IT department and the remaining work in the Marketing department. Four percent of the men work in the HR department and the remaining work in the Marketing department.
What is the ratio of the number of men working in the HR department to that of the women working in the same?
Correct
Solution (c)
The number of men working in the HR department = 13
The number of women working in HR department = 78
Required Ratio = 13:78 = 1:6
Incorrect
Solution (c)
The number of men working in the HR department = 13
The number of women working in HR department = 78
Required Ratio = 13:78 = 1:6
-
Question 5 of 5
5. Question
An office consists of 520 employees working in different departments HR, IT, Production and Marketing. The ratio of men to women in the organization is 5:3. 20 per cent of the men work in the IT department. 40 per cent of the women work in the HR department. The total number of employees in the Production department is 135. 40 percent of the men work in the Production department. Two-fifths of the women work in the IT department and the remaining work in the Marketing department. Four percent of the men work in the HR department and the remaining work in the Marketing department.
What is the number of women working in the Marketing department?
Correct
Solution (b)
No. of women working in Marketing Department is 34
Incorrect
Solution (b)
No. of women working in Marketing Department is 34












